In civil engineering surveying, bearing is very important to get the direction of a survey line. The whole circle bearing (W.C.B) is a common notation system of bearings. The definition of the whole circle bearing along with the measuring formula and example is discussed below.
What is Whole Circle Bearing (W.C.B)?
The horizontal angle made by a line with the magnetic north in the clockwise direction is the whole circle bearing of the line. This system is also known as the azimuthal system. In this system, only the north direction is used as reference meridian.
Whole Circle Bearing Formula
In whole circle bearing, the value of the bearing varies from 0° to 360°. Just measure the angle between true north line to the survey line to find the WCB. Don't forget to measure the clockwise angle in determining the whole circle bearing.
If a survey line falls in the first quadrant, its WCB varies from 0° to 90°. Similarly, in the second quadrant, WCB value varies from 90° to 180°; in the third quadrant, WCB value varies from 180° to 270°; in the fourth quadrant, WCB value varies from 270° to 360°.
Whole Circle Bearing Example
To understand the whole circle bearing measurement clearly, please carefully observe the following image illustrations.
{adselite}

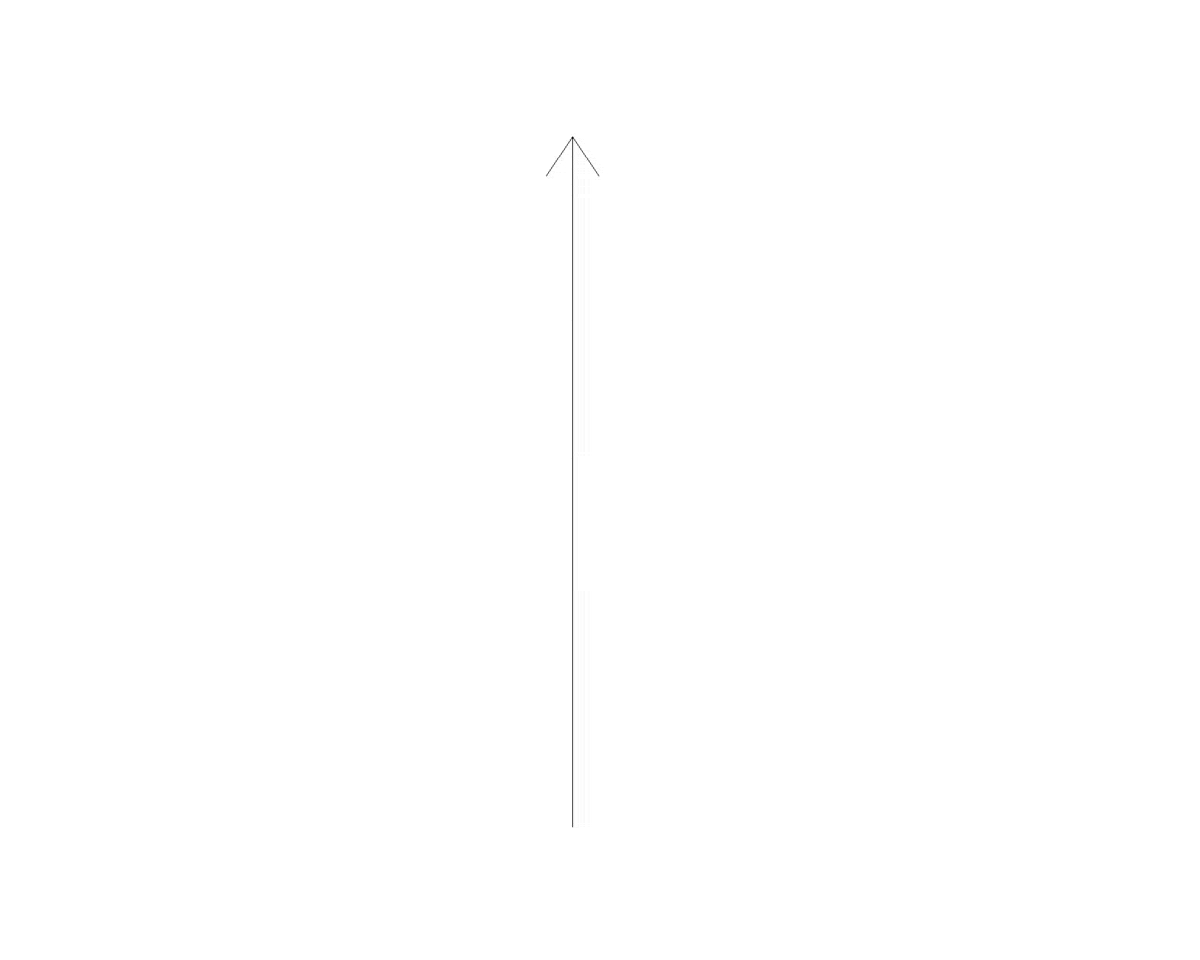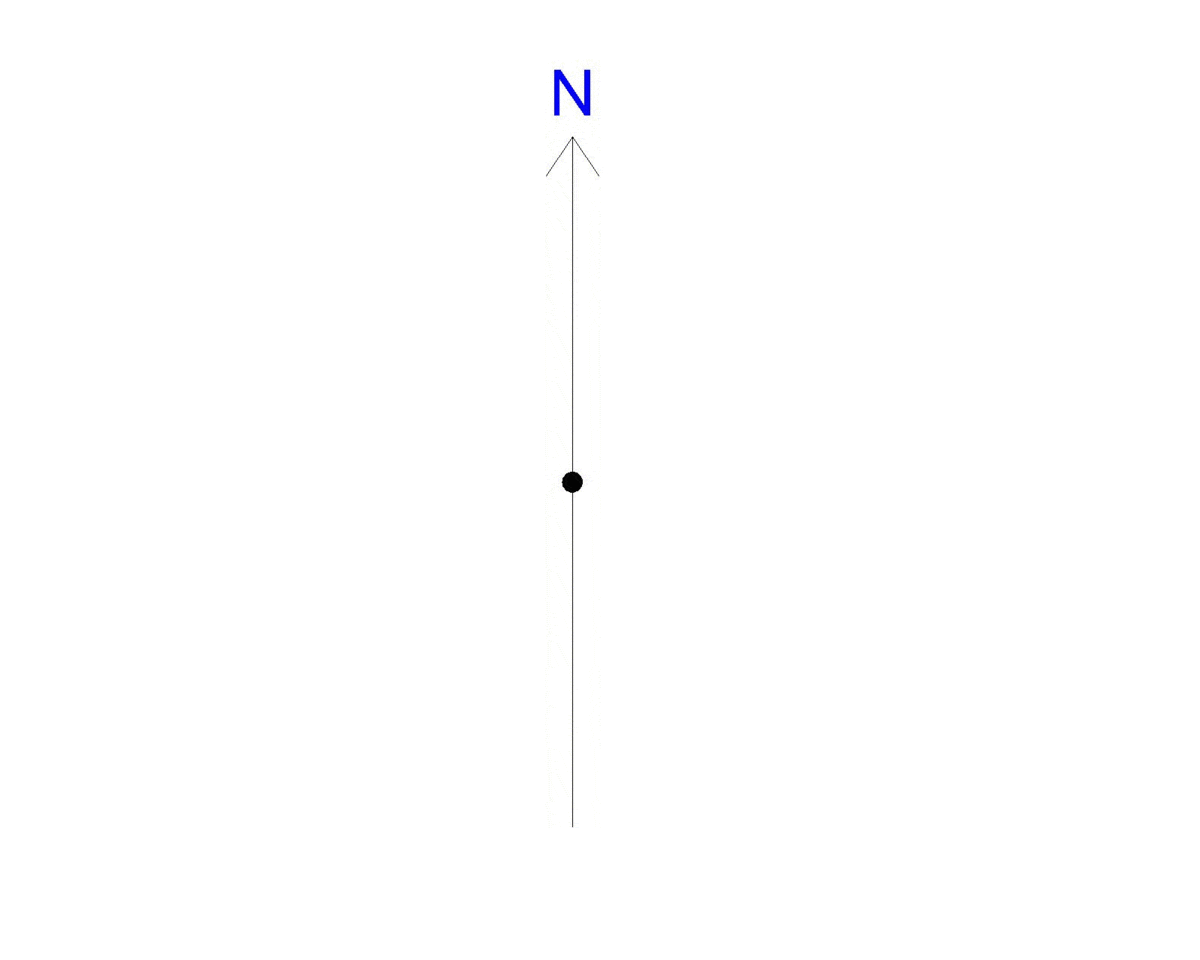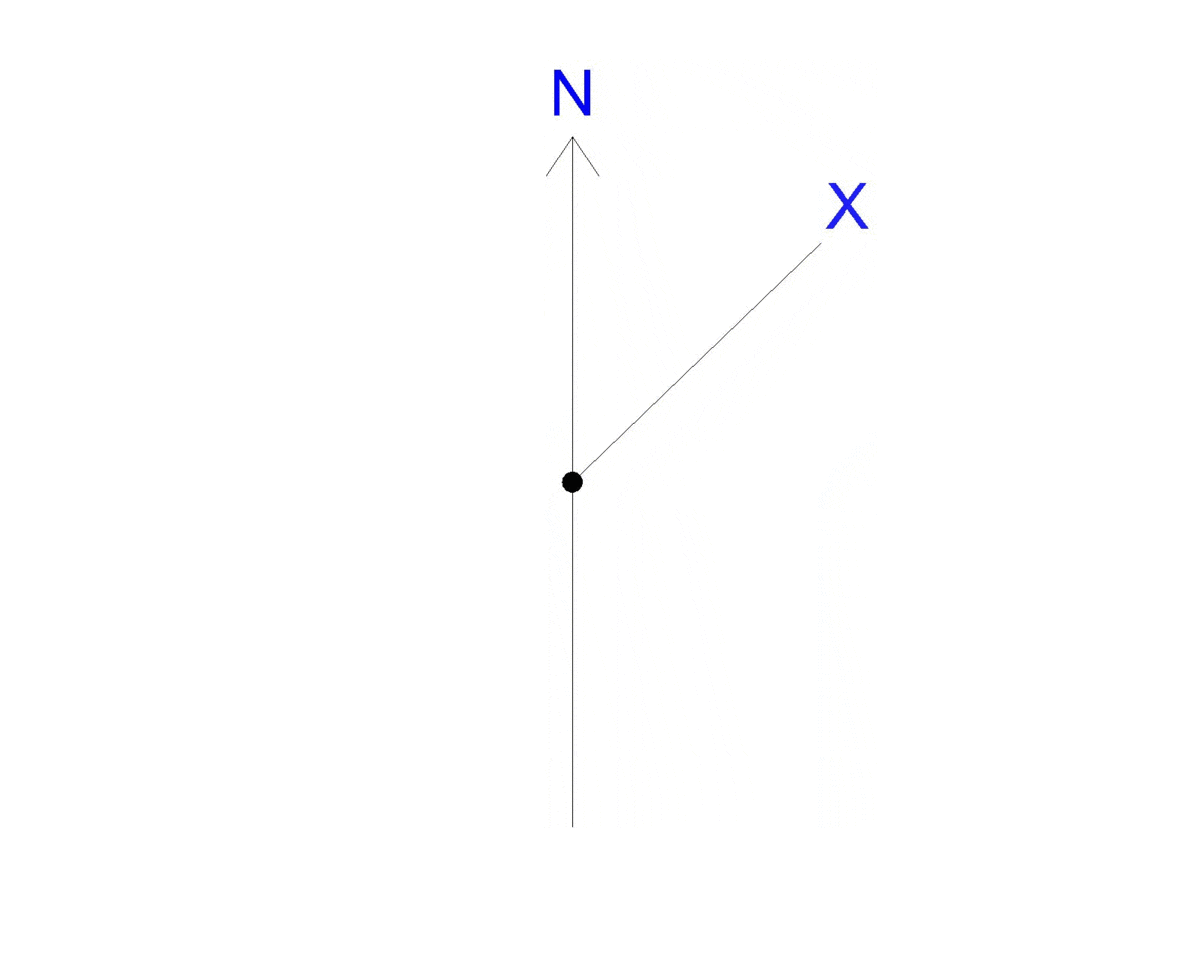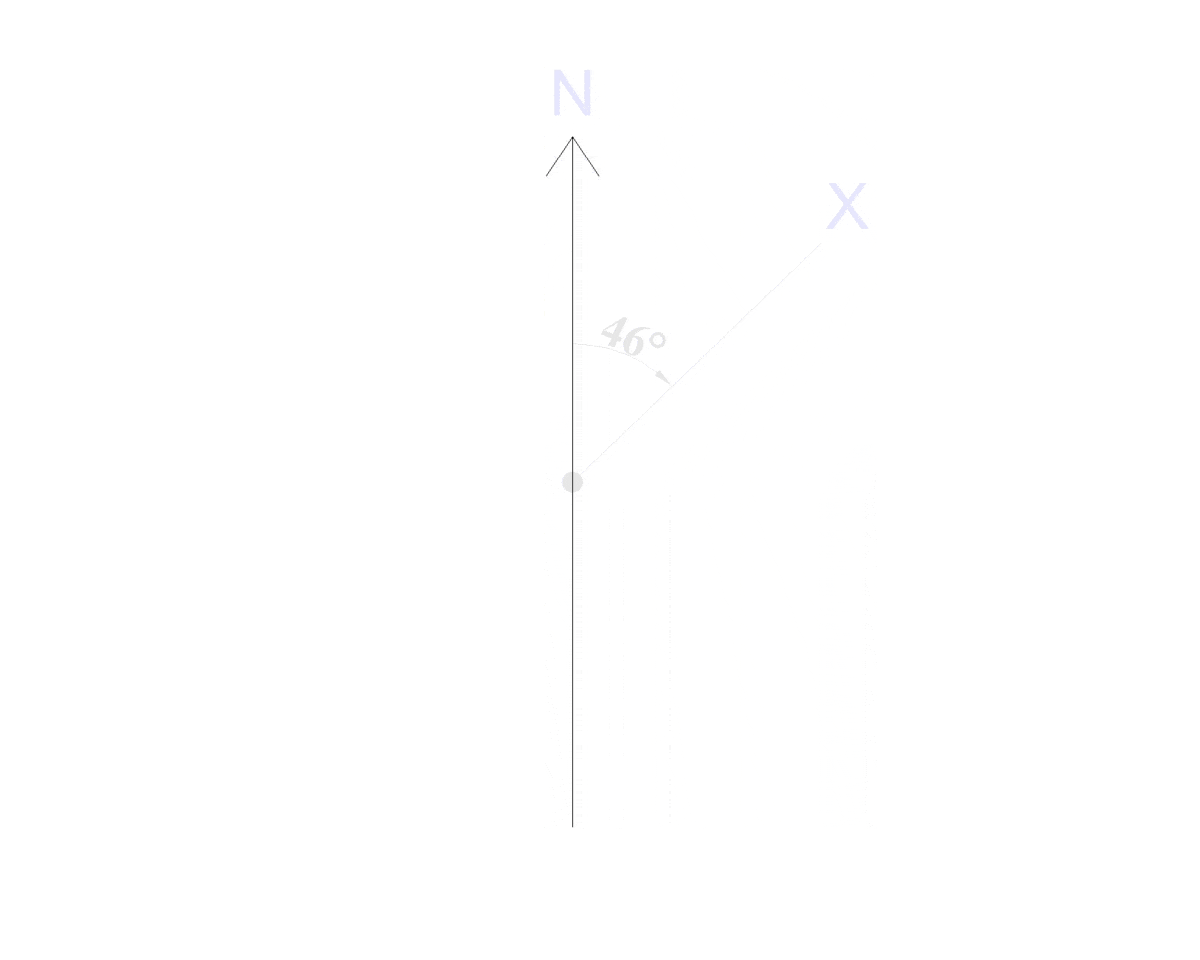
In the above images, the vertical line N is indicating the north line. In Fig 1, line X is in the first quadrant. It is at an angle of 46° with the north line in the clockwise direction. Therefore, whole circle bearing (W.C.B) of line X is 46°.
On the other hand, the line Y in Fig 2, is making 226° angle with the north line in the clockwise direction. So, whole circle bearing (W.C.B) of line Y is 226°.

